Calculation
First of all, let’s assume that a target gene concentration has been estimated together with its relative uncertainty, by applying the Poisson law as explained in the item [Poisson Law application].
Relative Uncertainty
Depending on the target concentration value in the well, the Relative Uncertainty forms a U-Curve which is made of 2 asymptotic curves:
- The sampling error curve, occurring at low target concentrations (on the left side in the [Figure A] below);
- The quantification error curve, occurring at high target concentrations (on the right side in the [Figure A] below).
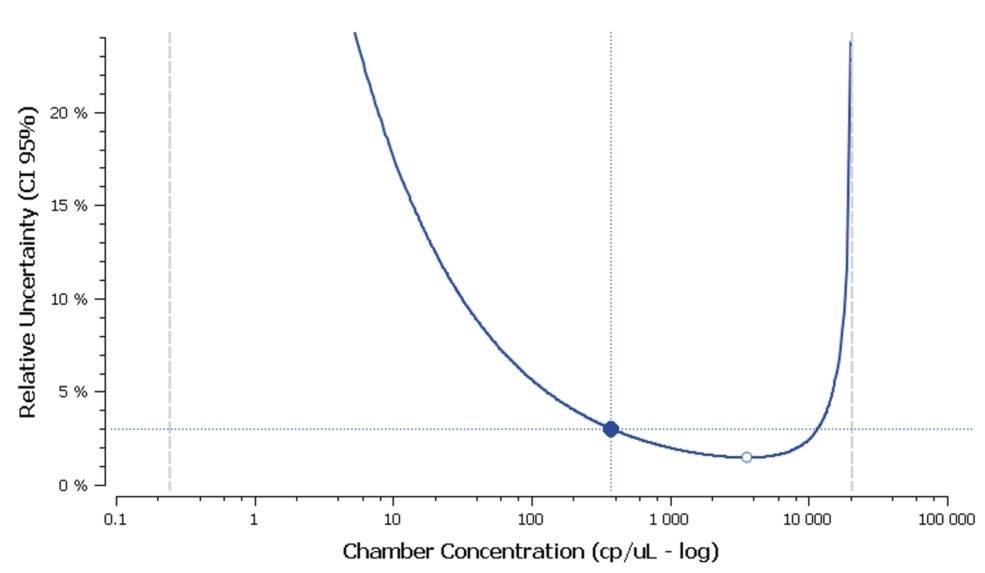
in a well that includes 28 000 partitions (in logarithmic view).
Sampling Error
The sampling error can be approximated as follows:
CUsampling∼((zc)/sqrt(C0V))
where V is the total partition volume, C0 is the well concentration, and zc = 1.96 for a confidence level of 95%.
- Note that, whatever the total number of partitions N and the confidence level zc the relative uncertainty is always minimized at p/N∼79.7, which corresponds to the white point lying on the U-Curve in the [Figure 1] above.
Minimal & Maximal Limits of Detection
The Minimal & Maximal Limits of Detection are represented by 2 vertical lines framing the U-Curve:
- The Minimal Limit of Detection LODminLODmin at 95% (left vertical dotted line in the [Figure 1] above) is the smallest target concentration in the well for which probability of having at least 1 positive partition is more than 95%. If the Limit of Blank is zero, we have:
LODmin = 3/V
- The Maximal Limit of Detection LODmax at 95% (right vertical dotted line in the [Figure A] above) is the highest target concentration in the well for which probability of having at least 1 negative partition is more than 95%) for N ranging from 10,000 to 1,000,000:
LODmax∼10 N/V
- The Dynamic range of Detection DrD at 95% is the number of decades from LODmin to LODmax at 95%. If the Limit of Blank is zero, we have:
DrD∼log10(N)+0.5
- The Minimal & Maximal Limits of Quantification are defined with respect to a maximal relative uncertainty Umax which is considered as acceptable for the current digital PCR experiment:
- The Minimal Limit of Quantification \(LOQ_{min}\) at 95% is the smallest target concentration in the well for which the relative uncertainty at 95% is smaller than Umax .Its value is given by the first intersection point of the 95% U-Curve and the horizontal line y = Umax (which corresponds to the blue point lying on the U-Curve in the [Figure 1] above).
- The Maximal Limit of Quantification LOQmax at 95% is the highest target concentration in the well for which the relative uncertainty at 95% is larger than Umax Its value is given by the second intersection point of the 95% U-Curve and the horizontal line y = Umax (see the [Figure 1] above).
Dynamic Range of Quantification
Finally, the Dynamic range of Quantification DrQ associated with an acceptable uncertainty value Umax is given by this formula:
DrQ(Umax) = LOQmax(Umax) − LOQmin(Umax)
Want to discover more dPCR experiments?
Check out our other tutorials!
